Unit 13 Competency 1 - Investigate and summarize the principles of thermodynamics
Suggested Objective a: Define and differentiate between heat, energy, entropy, and temperature
What is Heat?
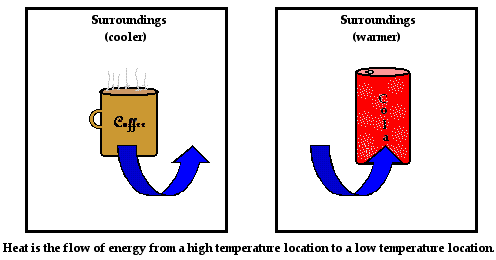
Consider a very hot mug of coffee on the countertop of your kitchen. For discussion purposes, we will say that the cup of coffee has a temperature of 80°C and that the surroundings (countertop, air in the kitchen, etc.) has a temperature of 26°C. What do you suppose will happen in this situation? I suspect that you know that the cup of coffee will gradually cool down over time. At 80°C, you wouldn't dare drink the coffee.Even the coffee mug will likely be too hot to touch. But over time, both the coffee mug and the coffee will cool down. Soon it will be at a drinkable temperature. And if you resist the temptation to drink the coffee, it will eventually reach room temperature. The coffee cools from 80°C to about 26°C. So what is happening over the course of time to cause the coffee to cool down? The answer to this question can be both macroscopic and particulate in nature.
On the macroscopic level, we would say that the coffee and the mug are transferring heat to the surroundings. This transfer of heat occurs from the hot coffee and hot mug to the surrounding air. The fact that the coffee lowers its temperature is a sign that the average kinetic energy of its particles is decreasing. The coffee is losing energy. The mug is also lowering its temperature; the average kinetic energy of its particles is also decreasing. The mug is also losing energy. The energy that is lost by the coffee and the mug is being transferred to the colder surroundings. We refer to this transfer of energy from the coffee and the mug to the surrounding air and countertop as heat. In this sense, heat is simply the transfer of energy from a hot object to a colder object.
Now let's consider a different scenario - that of a cold can of pop placed on the same kitchen counter. For discussion purposes, we will say that the pop and the can which contains it has a temperature of 5°C and that the surroundings (countertop, air in the kitchen, etc.) has a temperature of 26°C. What will happen to the cold can of pop over the course of time? Once more, I suspect that you know the answer. The cold pop and the container will both warm up to room temperature. But what is happening to cause these colder-than-room-temperature objects to increase their temperature? Is the cold escaping from the pop and its container? No! There is no such thing as the cold escaping or leaking. Rather, our explanation is very similar to the explanation used to explain why the coffee cools down. There is a heat transfer.
Over time, the pop and the container increase their temperature. The temperature rises from 5°C to nearly 26°C. This increase in temperature is a sign that the average kinetic energy of the particles within the pop and the container is increasing. In order for the particles within the pop and the container to increase their kinetic energy, they must be gaining energy from somewhere. But from where? Energy is being transferred from the surroundings (countertop, air in the kitchen, etc.) in the form of heat. Just as in the case of the cooling coffee mug, energy is being transferred from the higher temperature objects to the lower temperature object. Once more, this is known as heat - the transfer of energy from the higher temperature object to a lower temperature object.
The universe is made up of matter and energy. Matter — anything that has mass and takes up space — is pretty straightforward and easy![]() to grasp, but energy is a bit more abstract.
to grasp, but energy is a bit more abstract.
In physics, energy
Links to an external site. is the ability to do work, or the ability to move or elicit change![]() in matter. In effect, the amount of energy something has refers to its capacity to cause things to happen.
in matter. In effect, the amount of energy something has refers to its capacity to cause things to happen.
Energy has a few important properties. For one, energy is always "conserved" — it cannot be created or destroyed. It can, however, be transferred between objects or systems by the interactions of forces. For example, the energy in vegetables is transferred to the people who digest![]() them.
them.
nother property of energy is that it comes in multiple forms, and can be converted from one form to another. The two most common or basic forms of energy are kinetic energy and potential energy.
Kinetic energy is the energy of motion. A ball has kinetic energy as it flies through the air — it has the ability to do work in that it can act upon other objects with which it collides.
Potential energy is a kind of stored energy that objects have because of their position or configuration. A cup on a table has potential energy; if you knock the cup off the table, gravity will accelerate![]() the cup, and its potential energy will convert to kinetic energy. A stressed bow also has potential energy.
the cup, and its potential energy will convert to kinetic energy. A stressed bow also has potential energy.
Many other types of energy exist, including electrical, chemical, thermal, electromagnetic and nuclear Links to an external site..
In the early 20th century, scientists theorized that mass and energy are intimately linked. Albert Einstein Links to an external site. described this so-called mass-energy equivalence with his famous equation, E = mc2, in which "E" stands for "energy," "m" denotes "mass" and "c" is the speed of light.
Information is copied from http://www.livescience.com/42881-what-is-energy.html Links to an external site.on December 12, 2014.
What Is Energy REALLY?!
Links to an external site.
What is entropy?
The word entropy is sometimes confused with energy. Although they are related quantities, they are distinct.
As described in previous sections, energy measures the capability of an object or system to do work.
Entropy, on the other hand, is a measure of the "disorder" of a system. What "disorder refers to is really the number of different microscopic states a system can be in, given that the system has a particular fixed composition, volume, energy, pressure, and temperature. By "microscopic states", we mean the exact states of all the molecules making up the system.
The idea here is that just knowing the composition, volume, energy, pressure, and temperature doesn't tell you very much about the exact state of each molecule making up the system. For even a very small piece of matter, there can be trillions of different microscopic states, all of which correspond to the sample having the same composition, volume, energy, pressure, and temperature. But you're ignorant of exactly which one the system is in at any given moment - and that turns out to be important.
Why should it be important, after all, if you know the bulk properties. Isn't that all one usually needs? It turns out that no, in fact if you want to, say, exact energy from say steam and convert it to useful work, those details turn out to be crucial! (More on this below).
For those that are technically inclined, the exact definition is
Entropy = (Boltzmann's constant k) x logarithm of number of possible states
= k log(N).
Since the logarithm of a number always increases as the number increases, we see that the more possible states that the system can be in (given that it has a particular volume, energy, pressure, and temperature), then the greater the entropy.
Again, because we can't see which particular microscopic state a system is in, people often like to say that entropy is quantitative measure of how uncertain or ignorant one is about the exact, detailed, microscopic state of a system. Or, another popular way of saying this is that entropy measures the microscopic disorder of a system.
As a simple example, suppose that you put a marble in a large box, and shook the box around, and you didn't look inside afterwards. Then the marble could be anywhere in the box. Because the box is large, there are many possible places inside the box that the marble could be, so the marble in the box has a high entropy. Now suppose you put the marble in a tiny box and shook up the box. Now, even though you shook the box, you pretty much know where the marble is, because the box is small. In this case we say that the marble in the box has low entropy.
The same idea applies to the arrangements of atoms of a gas in a jar at room temperature. The smaller the jar, the lower the entropy. But keep in mind that we also have to consider the velocities of the gas particles to have full knowledge of their states. The higher the temperature of the gas, the faster the gas particles are moving on average, so the wider the range of possible velocities for the gas particles, and hence, the more uncertainty we have about the velocity of any particular particle. Thus, higher temperature, as well as greater volume, mean higher entropy.
Scientists say that entropy, like energy, volume, temperature, and pressure, is another thermodynamic state variable of a system. It turns out that, for a simple system, if you know any two of these state variables, then the others are all determined. Although the word entropy might seem like a mysterious concept, its really not. Remember that its really just a measure of the number states a system can be in, given the constraints on the system.
What is entropy good for? Knowing the entropy of a system can tell us many things about what can and can't happen. In particular, its the basis for the second law of thermodynamics: the Universe evolves such that its total entropy always stays the same or increases (The first law of thermodynamics is conservation of energy).
Why is this so? In fact, the basic idea of entropy is simple to understand. Suppose you are floating out in space and you have a jar containing a particular gas, say argon. When you open the jar for a moment, the argon will almost certainly escape out into space. After the argon has escaped, its entropy is greatly increased (and it continues to increase as the gas expands). How do I know that the entropy increased? This is because the number of states that the argon gas can be in when it occupies a much larger volume is much greater than when its confined to the jar. So, the entropy of the gas increases when the argon escapes. But why must the argon escape? Well, in fact, prior to opening the jar, if you arranged the microscopic states of the argon molecules in just the right way, you could open the jar for a moment and not have the argon escape. The point is that it is highly improbable that the argon is in one of these special non-escaping states when you open the jar - most of the states lead to the gas escaping. This is really the content of the second law - that if you begin not knowing the microscopic state of a system, then the system is more than likely to evolve to state where you are even more ignorant of its exact microscopic state. Just knowing the thermodynamic state variables of a system, such as its temperature and pressure, means you are in fact ignorant about the initial exact microscopic state - all you can know from the state variables is the number of possible microscopic states it can be in, i.e. the entropy. Hence, for most situations we encounter, chances are that entropy will increase with time.
It is very interesting to compare the behavior of entropy compared to energy. Unlike energy, entropy can be created (but not generally destroyed). In fact, your body is creating some right now as it generates heat. One of the reasons that your body temperature has to be higher than the surrounding air, or that you have to sweat off water if it isn't, is that you have to get rid of the extra entropy (otherwise, you would become disorganized and eventually die). The energy that your warm body radiates carries away the extra entropy. It does this because losing this energy decreases the number of microscopic states that the atoms and molecules of your body can be in.
Another practical example of entropy is the following. Suppose we want to use a source of heat, say, from steam generated by heating water, to drive some kind of turbine. Then, it turns out, by considering entropy, that the maximum efficiency of our process will be less than 100%. The reason that this is so is because when heat is brought into the turbine, it carries with it some entropy. We can't keep this entropy in the turbine, because the turbine would become microscopically disordered and eventually break. So, some heat energy has to be released to the outside world to get rid of this entropy to protect the turbine. The heat released for this purpose therefore can't be converted into work (otherwise it wouldn't be available anymore to release as heat). We get rid of the unwanted entropy by rejecting this heat to the outside world at a lower temperature than we brought the heat in at. The reason for the lower temperature is that the heat released into a low temperature environment carries out more entropy from the turbine than the entropy this same amount of heat carries into the turbine at a high temperature. This is because heat disrupts a cold system more than a hot one, because the hot one is already more disordered. Thus, we must only sacrifice some of the heat carried into the turbine to get rid of the entropy imported into the turbine by that heat in the first place. One can see from this discussion, however, why power plants need a cold temperature environment to dump their waste heat.
Now this all might seem a little too abstract. Here's another way to look at it: The kinetic energy of the steam molecules is large (because the steam is hot), but the directions of the molecules are disordered. Somehow, to convert all of the energy of the steam into useful work, you'd have to line them all up in the same direction (at least, say, one at a time or in groups). But you're ignorant of the exact configuration at any given instant, right? And even if you weren't, how are you going to get in there and actually do it for each molecule? Clearly, the microscopic disorder is a barrier. This shows why being ignorant of those details might seem minor intuitively, but actually has real consequences for real things you would like to do!
This example above demonstrates how heat energy, because it can't be completely converted to mechanical energy in a turbine, is, in a sense, of lesser quality than mechanical energy. People have in fact rated the quality of energy in this sense for many different sources. Solar electric energy captured by photovoltaic cells, in particular, is energy of very high "quality". Virtually all of it can be converted to mechanical energy.
Entropy can also be applied to many other situations. For example, it can be used to predict the direction that a chemical reaction will proceed.
Information copied from http://www.nmsea.org/Curriculum/Primer/what_is_entropy.htm Links to an external site.on December 12, 2014.
What Is Entropy?
Links to an external site.
Suggested Objective b: Examine how heat energy is transferred from higher temperature to lower temperature until equilibrium is reached
Thermal Equilibrium
In the discussion of the cooling of the coffee mug, the countertop and the air in the kitchen were referred to as the surroundings. It is common in physics discussions of this type to use a mental framework of a system and the surroundings. The coffee mug (and the coffee) would be regarded as the system and everything else in the universe would be regarded as the surroundings. To keep it simple, we often narrow the scope of the surroundings from the rest of the universe to simply those objects that are immediately surrounding the system. This approach of analyzing a situation in terms of system and surroundings is so useful that we will adopt the approach for the rest of this chapter and the next.
Now let's imagine a third situation. Suppose that a small metal cup of hot water is placed inside of a larger Styrofoam cup of cold water. Let's suppose that the temperature of the hot water is initially 70°C and that the temperature of the cold water in the outer cup is initially 5°C. And let's suppose that both cups are equipped with thermometers (or temperature probes) that measure the temperature of the water in each cup over the course of time. What do you suppose will happen? Before you read on, think about the question and commit to some form of answer. When the cold water is done warming and the hot water is done cooling, will their temperatures be the same or different? Will the cold water warm up to a lower temperature than the temperature that the hot water cools down to? Or as the warming and cooling occurs, will their temperatures cross each other?
Fortunately, this is an experiment that can be done and in fact has been done on many occasions. The graph below is a typical representation of the results.
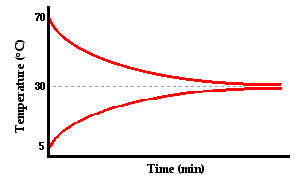
As you can see from the graph, the hot water cooled down to approximately 30°C and the cold water warmed up to approximately the same temperature. Heat is transferred from the high temperature object (inner can of hot water) to the low temperature object (outer can of cold water). If we designate the inner cup of hot water as the system, then we can say that there is a flow of heat from the system to the surroundings. As long as there is a temperature difference between the system and the surroundings, there is a heat flow between them. The heat flow is more rapid at first as depicted by the steeper slopes of the lines. Over time, the temperature difference between system and surroundings decreases and the rate of heat transfer decreases. This is denoted by the gentler slope of the two lines. Eventually, the system and the surroundings reach the same temperature and the heat transfer ceases. It is at this point, that the two objects are said to have reached thermal equilibrium.
Information copied from http://www.physicsclassroom.com/class/thermalP/Lesson-1/What-is-Heat Links to an external site. on December 12, 2014
What Is Heat Transfer?
Links to an external site.
Suggested Objective c: Examine the laws of thermodynamics (Zeroth, First, Second, and Third)
In our chapter on electric circuits Links to an external site., we learned that a difference in electric potential between two locations causes a flow of charge along a conducting path between those locations. As long as an electric potential difference Links to an external site. is maintained, a flow of charge will exist. Now in this chapter we learn a similar principle related to the flow of heat. A temperature difference between two locations will cause a flow of heat along a (thermally) conducting path between those two locations. As long as the temperature difference is maintained, a flow of heat will occur. This flow of heat continues until the two objects reach the same temperature. Once their temperatures become equal, they are said to be at thermal equilibrium and the flow of heat no longer takes place.
This principle is sometimes referred to as the zeroeth law of thermodynamics. This principle became formalized into a law after the first, second and third laws of thermodynamics had already been discovered. But because the law seemed more fundamental than the previously discovered three, it was titled the zeroeth law. All objects are governed by this law - this tendency towards thermal equilibrium. It represents a daily challenge for those who wish to control the temperature of their bodies, their food, their drinks and their homes. We use ice and insulation to try to keep our cold drinks cold and we use insulation and ongoing pulses of microwave energy to keep our hot drinks hot. We equip our vehicles, our homes and our office buildings equipped with air conditioners and fans in order to keep them cool during the warm summer months. And we equip these same vehicles and buildings with furnaces and heaters in order to keep them warm during the cold winter months. Whenever any of these systems are at a different temperature as the surroundings and not perfectly insulated from the surroundings (an ideal situation), heat will flow. This heat flow will continue until the system and surroundings have achieved equal temperatures. Because these systems have a considerably smaller volume than the surroundings, there will be a more noticeable and substantial change in temperature of these systems.
Information copied from http://www.physicsclassroom.com/class/thermalP/Lesson-1/What-is-Heat Links to an external site. on December 12, 2014
HeatHeat may be defined as energy in transit from a high temperature Links to an external site. object to a lower temperature object. An object does not possess "heat"; the appropriate term for the microscopic energy in an object is internal energy Links to an external site.. The internal energy may be increased by transferring energy to the object from a higher temperature (hotter) object - this is properly called heating. 
|
Index
Links to an external site. Heat engine concepts Links to an external site. |
||
|
Go Back |
Heat and Work Example
This example of the interchangeability of heat Links to an external site. and work Links to an external site. as agents for adding energy to a system can help to dispel some misconceptions about heat. I found the idea in a little article by Mark Zemansky entitled "The Use and Misuse of the Word 'Heat' in Physics Teaching". One key idea from this example is that if you are presented with a high temperature Links to an external site. gas, you cannot tell whether it reached that high temperature by being heated, or by having work done on it, or a combination of the two. To describe the energy that a high temperature object has, it is not a correct use of the word heat to say that the object "possesses heat" - it is better to say that it possesses internal energy Links to an external site. as a result of its molecular motion. The word heat is better reserved to describe the process of transfer of energy from a high temperature object to a lower temperature one. Surely you can take an object at low internal energy and raise it to higher internal energy by heating it. But you can also increase its internal energy by doing work on it, and since the internal energy of a high temperature object resides in random motion of the molecules, you can't tell which mechanism was used to give it that energy. In warning teachers and students alike about the pitfalls of misusing the word "heat", Mark Zemansky advises reflecting on the jingle:
Zemansky points to the First Law of Thermodynamics Links to an external site. as a clarifying relationship. The First Law identifies both heat and work as methods of energy transfer which can bring about a change in the internal energy of a system. After that, neither the words work or heat have any usefulness in describing the final state of the sytem - we can speak only of the internal energy of the system.
|
Index
Links to an external site. Internal energy concepts Links to an external site. Reference Zemansky Links to an external site. |
|||||
|
Go Back |
Mechanical Equivalent of HeatHeat Links to an external site. flow and work Links to an external site. are both ways of transferring energy Links to an external site.. As illustrated in the heat and work example Links to an external site., the temperature Links to an external site. of a gas can be raised either by heating it, by doing work on it, or a combination of the two. In a classic experiment in 1843, James Joule showed the energy equivalence of heating and doing work by using the change in potential energy Links to an external site. of falling masses to stir an insulated container of water with paddles. Careful measurements showed the increase in the temperature of the water to be proportional to the mechanical energy used to stir the water. At that time calories were the accepted unit of heat and joules became the accepted unit of mechanical energy. The British Thermal Unit was also introduced. Their relationship to joules is 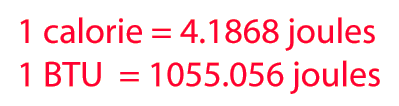
These conversions are the International Steam Table (IT) values, and variations up to 0.5% will be found since they were originally based on energy for temperature changes in water, and that varies a small amount based on what temperature of water is used to establish it. The heat capacity of water does change slightly with temperature. |
The above information has various links to click for additional information. Feel free to click the links to learn more. Take notes as needed. The site also has at the bottom of the page an exercise to help you with learning to calculate temperature. Information was copied from http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/heat.html Links to an external site. on December 12, 2014.
What is Temperature?
|
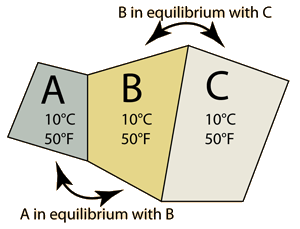
One approach to the definition of temperature is to consider three objects, say blocks of copper, iron and alumninum which are in contact such that they come to thermal equilibrium Links to an external site.. By equilibrium we mean that they are no longer transferring any net energy to each other. We would then say that they are at the same temperature, and we would say that temperature is a property of these objects which implies that they will no longer transfer net energy to one another. We could say that A is at the same temperature as C even though they are not in contact with each other. This scenario is called the "zeroth law of thermodynamics" since this understanding logically precedes the ideas contained in the important First Links to an external site. and Second Links to an external site. Laws of Thermodynamics. |
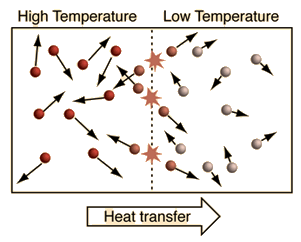 Kinetic temperature 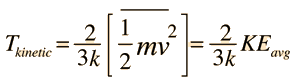
Ideas to think about:
|
An important idea related to temperature is the fact that a collision between a molecule with high kinetic energy Links to an external site. and one with low kinetic energy will transfer energy to the molecule of lower kinetic energy. Part of the idea of temperature is that for two collections of the same type of molecules that are in contact with each other, the collection with higher average kinetic energy will transfer energy to the collection with lower average kinetic energy. We would say that the collection with higher kinetic energy has a higher temperature, and that net energy transfer will be from the higher temperature collection to the lower temperature collection, and not vice versa. Clearly, temperature has to do with the kinetic energy of the molecules, and if the molecules act like independent point masses, then we could define temperature in terms of the average translational kinetic energy of the molecules, the so-called "kinetic temperature Links to an external site.". The average kinetic energy of the molecules of an object is an important part of the concept of temperature and provides some useful intuition about what temperature is. If all matter just consisted of independently moving point masses that just experiencedelastic collisions Links to an external site. with each other, that would be an adequate picture of temperature. |
|
Internal or coordinated motions of molecules complicate the picture of temperature. |
Molecules for materials other than monoatomic noble gases like helium have the possibility of energy other than the translational kinetic energy of point masses. Molecules can have rotational and translational kinetic energy and the molecules in periodic solids can have collective modes of motion that have energy. This complicates the idea of temperature because they affect the conditions under which energy would be transferred from one collection of molecules to another, and we want to hang onto the idea that if energy is spontaneously transferred from A to B, then A is at a higher temperature than B. |
|
Defining temperature in terms of entropy. |
Many of the most fundamental arguments in physics are those having to do withmultiplicity Links to an external site.. If there are more ways to accomplish a given state of a system of particles, then other states will spontaneously transition to that state over time if the transition is consistent with conservation of energy Links to an external site.. The multiplicity of a system of particles is stated in terms of its entropy Links to an external site.. Systems will spontaneously proceed toward states with higher entropy (2nd law of thermodynamics Links to an external site.). But what does that have to do with temperature? It turns out that if you have two systems A and B which are thermally coupled to each other, and you make a change of internal energy ΔU to each of them, then if A experiences a larger change in entropy S than B, then A is the lower temperature and energy will spontaneously transfer from B to A. This is far less intuitive than high-speed molecules hitting low-speed molecules and transferring energy to them! But with the variety of energy forms and collective modes in systems, it turns out to be a more reliable approach to temperature. See the examples where this approach with the ideal gas Links to an external site. andEinstein solid Links to an external site. leads you back to the more intuitive kinetic energy statements. |
Under conditions where the kinetic temperature Links to an external site. as derived from kinetic theory Links to an external site. provides reasonable accuracy, we perceive temperature as the average translational kinetic energy associated with the disordered motion of atoms and molecules. That makes it intuitive that the flow of heat is from a high temperature region toward a lower temperature region since higher energy molecules are striking lower energy molecules and transferring energy to them. Temperature is not directly proportional to internal energy Links to an external site. since temperature measures only the kinetic energy part of the internal energy, so two objects with the same temperature do not in general have the same internal energy (see water-metal example Links to an external site.). Temperatures are measured in one of the three standard temperature scales Links to an external site. (Celsius, Kelvin, and Fahrenheit).
| Suppose we are dealing with two equal mass objects at ordinary temperatures and can presume that kinetic temperature gives a reasonable description of their behavior. If the two objects are at the same temperature, then we would say that their average translational kinetic energies are the same. That does not imply that their total internal energies are the same, because the potential energies associated with intermolecular forces can be quite different. |  |
Even if there are internal kinetic energies other than translational KE, it could be that heat transfer is mainly by collisional transfer. In such cases this picture might help understand that just a portion of the total internal energy of objects is involved in the conditions for thermal equilibrium.
The above information has various links to click for additional information. Feel free to click the links to learn more. Take notes as needed. The site also has at the bottom of the page an exercise to help you with learning to calculate temperature. Information was copied from http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/temper.html Links to an external site. on December 12, 2014.
The 4 Laws
There are 4 laws to thermodynamics, and they are some of the most important laws in all of physics. The laws are as follows
- Zeroth law of thermodynamics – If two thermodynamic systems are each in thermal equilibrium with a third, then they are in thermal equilibrium with each other.
- First law of thermodynamics – Energy can neither be created nor destroyed. It can only change forms. In any process, the total energy of the universe remains the same. For a thermodynamic cycle the net heat supplied to the system equals the net work done by the system.
- Second law of thermodynamics – The entropy of an isolated system not in equilibrium will tend to increase over time, approaching a maximum value at equilibrium.
- Third law of thermodynamics – As temperature approaches absolute zero, the entropy of a system approaches a constant minimum.
Before I go over these laws in more detail, it will be easier if I first introduce Entropy.
Entropy and Phase Space
Entropy is a very important thing in the realm of thermodynamics. It’s the core idea behind the second and third laws and shows up all over the place. Essentially entropy is the measure of disorder and randomness in a system. Here are 2 examples
- Let’s say you have a container of gas molecules. If all the molecules are in one corner then this would be a low entropy state (highly organised). As the particle move out and fill up the rest of the container then the entropy (disorder) increases.
- If you have a ball flying through the air then it will start off with its energy organised i.e. the kinetic energy of motion. As it moves through the air however, some of the kinetic energy is distributed to the air particles so the total entropy of system has increased (the total energy is conserved however, due to the first law)
To get a more detailed picture of entropy we need to look at the concept of Phase Space. Some of the concepts for this may be a bit confusing but bear with me, once you’ve got your head round it it’s not that bad.
A phase space is just like a graph, but a point on this graph represents the whole state of a system. Let’s use an example. Imagine I have a box with 4 gas particles inside. Each point in the phase space for this system tells you where all 4 balls are located in the box.

In our example we are only interested in the positions of the 4 particles, so each point in phase space must contain an x, y, and z co-ordinate for each particle so our phase space is 3N dimensional, where N is the number of particles in the system. So in our case, the phase space is 12 dimensional, in order that each point can describe the location of 4 bodies.
In all the diagrams I will depict the phase space as 2D to make it easier to convey what it actually represents. For our purposes we will not need to consider the dimensions.
If we imagine that each of the particles is a different colour so we can keep track of their positions easier. If we imagine the case where all of the particles are located in one corner of the container then we have the situation

In terms of the system, there are multiple other combinations of the 4 particles that will be as organised as the above state

and so on. Each of these set-ups will correspond to a different position in phase space as they are all different layouts of the system of the 4 particles. If we add these to the phase space along with the original we get something like

These 5 layouts of the 4 particles, along with the 11 other combinations, make up a set of states that are (apart from the colours) indistinguishable. So in the phase space we could put a box around the 16 states that defines all the states inside it as being macroscopically indistinguishable.

The total phase space of a system will have many regions all of different shapes and sizes and could look like the following

But how is all this abstract representation linked to entropy. Entropy, given in equations as the symbol , is defined then as
Where is Boltzmann constant (
) and
is the volume of the box in phase space. All the points in a region of phase space have the same entropy, and the value of the entropy is related to the logarithm of the volume (originally Boltzmann never put the constant
in the formula as he wasn’t concerned with the units. The insertion of the k seemed to have come first from Planck).
Entropy can also be defined as the change when energy is transfered at a constant temperature
Where is the change in entropy,
is the energy or heat, and T is the constant temperature.
The Zeroth Law
The Zeroth law is so named as it came after the other 3. Laws 1, 2, and 3 had been around for a while before the importance of this law had been fully understood. It turned out that this law was so important and fundamental that it had to go before the other 3, and instead of renaming the already well known 3 laws they called the new one the Zeroth law and stuck it at the front of the list.
But what does it actually mean? The law states
“If two thermodynamic systems are each in thermal equilibrium with a third, then they are in thermal equilibrium with each other.”
Basically, if A=B and C=B then A=C. This may seem so obvious that is doesn’t need stating but without this law we couldn’t define temperature and we couldn’t build thermometers.
The First Law
The first law of thermodynamics basically states that energy is conserved; it can neither be created nor destroyed, just changed from one for to another,
“The total amount of energy in an isolated system is conserved.”
The energy in a system can be converted to heat or work or other things, but you always have the same total that you started with.
As an analogy, think of energy as indestructible blocks. If you have 30 blocks, then whatever you do to or with the blocks you will always have 30 of them at the end. You cant destroy them, only move them around or divide them up, but there will always be 30. Sometimes you may loose one or more, but they still have to be taken account of because Energy is Conserved.
Fundamental thermodynamic relation
From the second law we can write that the change in the internal energy, , or a system is equal to heat supplied to the system,
, minus any work done by the system,
,
From the definition of entropy above we can replace , and we can also make the replacement
giving us
Now if we have a system of particles that are different then we may get chemical reactions occuring, so we need to add one more term to take this into account
The Second Law
This is possibly the most famous (among scientists at least) and important laws of all science. It states;
“The entropy of the universe tends to a maximum.”
In other words Entropy either stays the same or gets bigger, the entropy of the universe can never go down.

The problem is, this sin’t always true. If you take our example of 4 atoms in a box then all of them being in one corner is a highly ordered system and so will have a low entropy, and then over time they’ll move around become more disordered and increasing the entropy. But there is nothing stopping them all randomly moving back to the corner. It’s incredible unlikely, but not actually impossible.
If you look at the problem in terms of phase space you can see that over time it’s more likely you’ll move into a bigger box, which means higher entropy, but there’s no actual barrier stopping you moving back into a smaller box.
The Third Law
The third law provides an absolute reference point for measuring entropy, saying that
“As the temperature of a system approaches absolute zero (−273.15°C, 0 K), then the value of the entropy approaches a minimum.”
The value of the entropy is usually 0 at 0K, however there are some cases where there is still a small amount of residual entropy in the system.
The Basics
When you heat something, depending on what it’s made of, it takes a different about of time to heat up. Assuming that power remains constant, this must mean that some materials require more energy to raise their temperature by 1K (1K is actually the same as 1°C, they just start at a different place. For more information click here) than others. If you think about it, this makes sense. A wooden spoon takes a lot longer to heat up than a metal one. We say that metal is a good thermal conductor and wood a poor thermal conductor. The energy required to raise 1kg of a substance by 1K is called it’s specific heat capacity. The formula we use to find how much energy is required to raise 1 kg of a substance by 1K is:
where = Energy,
= mass,
= specific heat capacity and
= change in temperature.
1a. Laura is cooking her breakfast before work on a Sunday morning (please send your sympathy messages that I had to work on a Sunday here). She doesn’t want to have to do any more washing up that is absolutely necessary, so decides to stir the spaghetti she is cooking with her fork, rather than have to wash a wooden spoon. She leaves the fork in the pan whilst she spreads her toast with margarine and grates some cheese. The stove provides 1000J of energy to the fork in the time she leaves it unattented. What would be the temperature increase in the fork, assuming half the energy provided will be lost to the surroundings and the initial temperature of the fork was 20°C, and the mass of the fork is 50g and is made of a material with a specific heat capacity of 460 Jkg-1K-1
Although I’m fairly sure I read somewhere that trying to work out energy changes in forks first thing in the morning was a symptom of insanity, it’s something I find myself doing from time to time. For this question, we’re going to need the equation Q=mcΔT this is an equation you’ll probably need a lot, so it’s worth trying to memorize it. It also springs up in chemistry too. First things first, we need to rearrange the equation to make ΔT the subject. Once you’ve rearranged this question you should get ΔT=Q/(mc). Substituting the values given to us in the question you get:
ΔT= 1000/(50 x 10-3 x 460)
ΔT= 43K
So since the initial temperature of the fork was 20°C, the final temperature of the fork would be 63°C.
Different Measures of Energy
- Internal Energy:
- Helmholtz free energy:
- Enthalpy:
- Gibbs Free Energy:
Maxwell’s Relations
Suggested Objective d: Examine temperature and thermal energy as related to molecular motion (conduction, convection, and radiation) and the states of matter (solid, liquid, and gas)
Heat and Temperature


 , is
, is  J/kg · ºC or 1 cal/g · ºC. That means it takes
J/kg · ºC or 1 cal/g · ºC. That means it takes  joules of heat to raise one kilogram of water by one degree Celsius. Substances that are easily heated, like copper, have a low specific heat, while substances that are difficult to heat, like rubber, have a high specific heat.
joules of heat to raise one kilogram of water by one degree Celsius. Substances that are easily heated, like copper, have a low specific heat, while substances that are difficult to heat, like rubber, have a high specific heat.
 is the change in temperature.
is the change in temperature.
|
||||||
 :
:
|
||||||
 is equal to the heat lost by the copper,
is equal to the heat lost by the copper, . We can set the heat gained by the gold to be equal to the heat lost by the copper, bearing in mind that the final temperature of the gold must equal the final temperature of the copper:
. We can set the heat gained by the gold to be equal to the heat lost by the copper, bearing in mind that the final temperature of the gold must equal the final temperature of the copper:
 and
and  tells us that the temperature change of the gold is equal to the temperature change of the copper. If the gold heats up by 30 Cº and the copper cools down by 30 Cº, then the two substances will reach thermal equilibrium at 50ºC.
tells us that the temperature change of the gold is equal to the temperature change of the copper. If the gold heats up by 30 Cº and the copper cools down by 30 Cº, then the two substances will reach thermal equilibrium at 50ºC.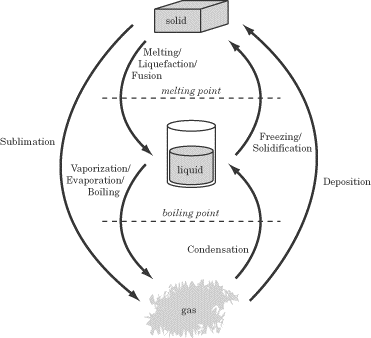
 J/kg. That means that you must add
J/kg. That means that you must add  J to change one kilogram of ice into water, and remove the same amount of heat to change one kilogram of water into ice. Throughout this phase change, the temperature will remain constant at 0ºC.
J to change one kilogram of ice into water, and remove the same amount of heat to change one kilogram of water into ice. Throughout this phase change, the temperature will remain constant at 0ºC. J/kg, meaning that you must add
J/kg, meaning that you must add  J to change one kilogram of water into steam, or remove the same amount of heat to change one kilogram of steam into water. Throughout this phase change, the temperature will remain constant at 100ºC.
J to change one kilogram of water into steam, or remove the same amount of heat to change one kilogram of steam into water. Throughout this phase change, the temperature will remain constant at 100ºC.
|
||||||





 , and a coefficient of volume expansion,
, and a coefficient of volume expansion,  . We can use these coefficients to determine the change in a substance’s length, L, or volume, V, given a certain change in temperature.
. We can use these coefficients to determine the change in a substance’s length, L, or volume, V, given a certain change in temperature.

|
||||||


 m. Because the brass expands more than the steel, the bimetallic strip will bend a little to compensate for the extra length of the brass.
m. Because the brass expands more than the steel, the bimetallic strip will bend a little to compensate for the extra length of the brass.Suggested Objective e: Investigate problems involving specific heat and heat capacity
We will investigate real-world problems involving heat and heat capacity in upcoming assignments. Have your notes handy to assist you with completing this work.









