Unit 11 Competency 1 - Examine fluid systems in engineering
Suggested Objective a: Examine how Netwon's laws of motion are applied in fluid systems
Please see this Quizlet Links to an external site. for terms you will need to know for fluid systems.
Fluid Systems
A fluid is any substance that cannot maintain its own shape. Fluid mechanics is concerned with the static and dynamics of fluid. Fluid static treats fluids in the equilibrium state. Fluid dynamics is concerned with fluid in motion relative to other parts. The following table summarizes the variables needed to define a fluid and its environment.
Variables Needed to Define Fluid
|
Quantity |
Symbol |
Object |
Unit |
|
Pressure |
p |
Scalar |
N/m2 |
|
Velocity |
v |
Vector |
m/s |
|
Density |
r |
Scalar |
kg/m3 |
|
Viscosity |
m |
Scalar |
kg.m |
|
Force |
F |
Vector |
N/kg |
|
Time |
t |
Scalar |
s |
Fluids is one of the oldest disciplines in physics and engineering. Ancient civilizations were faced with the difficult tasks of controlling water for agricultural development, water consumption, and travel. Agricultural developments led to the construction of irrigation channels, dams, weirs, pumps, and sprinkler systems. Human water consumption led to building wells, fountains, and water storage systems. The need for travel helped the development of ships and aeroplanes. Motion and behaviour of fluids is therefore critical to improving the quality of human life, even to the point of his survival.
Roots of fluids are extended to almost every aspect of science and engineering. Civil engineering, for example, is developed primarily from the need for fluid systems and structures. Mechanical engineering studies fluids in combustion, lubrication, and energy systems. Aeronautical engineering studies gas flow to produce energy and to provide lift on flying structures. Electrical engineering uses fluids to cool electronic devices with air flow. The study of fluids is essential for the chemical engineer, because the majority of chemical processing operations are conducted either partially or totally in the fluid phase. Flow processes in the human body, cardiac and cardiovascular systems, blood flow and respiratory system are few examples from the discipline of bio-fluid in the human body.
When an object is submerged in a fluid the fluid can only push on it, or compress it. The compression force is always a normal force, that is, it is always perpendicular to the surface of the object, independent of the orientation of the object. As shown in the following figure, the weight of the liquid exerts a force on the bottom of the container. This force produces a pressure on the bottom of the container. An important property of fluids is pressure.
Fluid Pressure
Pressure is the quantity which causes fluid flow or sustains the weight of a column of fluid. Fluid pressure is defined as the normal force exerted on a surface (real or imaginary) in a fluid per unit area. We define the pressure at a point in a fluid as the force (F) per unit area on a surface of area A
Also we can take a very small area and take the limit as the area goes to zero
If we replace the force in the above equation with the weight, we find that
Where m is the mass of the liquid in kg, A is the area of the bottom of the container in m2, and g is the acceleration of gravity m/s2. Note that the pressure is a scalar quantity; it does not have a direction. The SI unit for pressure is the Newton per square meter (N/m2), which is named pascal (Pa) after the French scientist Blaise Pascal (1623-1662). Two other commonly used pressure units are the bar, 1 bar = 100 kPa, and standard atmosphere, 1 atm = 101.325 kPa. The absolute pressure is measured relative to absolute vacuum. Most pressure-measuring devices, however, are calibrated to read zero in the atmosphere, and so they indicate the difference between the absolute zero pressure and the local atmospheric pressure. This difference is called the gauge pressure.
Information copied from http://www.g9toengineering.com/resources/fluidsystems.htm Links to an external site. on December 9, 2014
Suggested Objective b: Differentiate between mass and weight
The difference between mass Links to an external site.and weight Links to an external site. is that mass is the amount of matter Links to an external site. in a material and weight is a measure of how the force of gravity acts upon that mass.
Mass is the measure of the amount of matter in a body. Mass is denoted using m or M.
Weight is the measure of the amount of force acting on a mass due to the acceleration due to gravity. Weight usually is denoted by W. Weight is mass multiplied by the acceleration of gravity.
W = m * g
| Mass is a property of matter. The mass of an object is the same everywhere, | Weight depends on the effect of gravity. Weight varies according to location. |
| Mass can never be zero. | Weight can be zero if no gravity acts upon an object, as in space. |
| Mass does not change according to location. | Weight increases or decreases with higher or lower gravity. |
| Mass is a scalar quantity. It has magnitude. | Weight is a vector quantity. It has magnitude and is directed toward the center of the Earth or other gravity well. |
| Mass may be measured using an ordinary balance. | Weight is measured using a spring balance. |
| Mass usually is measured in grams and kilograms. | Weight often is measured in newtons, a unit of force. |
Information copied from http://chemistry.about.com/od/chemistryterminology/a/What-Is-The-Difference-Between-Mass-And-Weight.htm Links to an external site. on December 9, 2014
The Difference between Mass and Weight
Links to an external site.
Newton's Laws and Weight, Mass, and Gravity Links to an external site. (this site includes a video and quiz with plenty of information)
Newton's Laws: Weight, Mass And Gravity
Most of us have seen images of men walking on the moon. Now, even though the astronauts are wearing really heavy suits, they seem to bounce around the surface of the moon with very little effort. How is it that we can bounce around on the moon with ease while jumping here on Earth requires a lot of effort? The answer to this question lies within the difference between mass and weight.
Mass is a measure of how much matter an object contains, while weight is a measure of the force of gravity on the object. An object has the same composition, and therefore mass, regardless of its location. For example, a person with a mass of 70 kg on Earth has a mass of 70 kg in space as well as on the moon. However, that same person's weight is not the same since gravity is different in these locations. The person will weigh less on the moon because the moon has less gravity. To better understand the concepts of weight and mass, we must first consider gravity and its effect on objects.
What Is Gravity?
So what is gravity? Gravity is the attractive pull between two objects that have mass. The strength of gravity is directly proportional to the amount of mass of each object. In other words, the larger the objects, the greater the gravitational attraction between them. For example, the gravitational pull you experience on Earth is much greater than it would be on the moon because the Earth's mass is greater. An object with twice as much mass will exert twice as much gravitational pull on other objects.
 |
On the other hand, the strength of gravity is inversely related to the square of the distancebetween two objects. For example, if the distance between two objects doubles, meaning they're twice as far apart, the gravitational pull decreases by a factor of 4. This is because 2 squared is equal to 4. This means the effect of distance on gravitational attraction is greater than the effect of the masses of the objects.
Gravity As A Force
Gravity is a force. A force is simply a push or a pull experienced by objects that interact with each other. The interaction can be direct or at a distance, which is the case of gravity. Newton's laws tell us that if an unbalanced force acts on an object, it will change the object's state of motion. In other words, the object will accelerate. Since gravity is a force, gravity causes objects to accelerate.
Acceleration Due To Gravity
Let's look at an example of how gravity causes acceleration. If you drop a ball from a cliff, you will notice that the speed increases as it falls - it accelerates due to gravity. We have determined the acceleration of gravity is 9.8 m/sec^2 - that is, for free-falling objects on Earth. Free falling simply means no other forces, except gravity, are acting on the object. For example, any effect of wind resistance would be neglected. The velocity of a free-falling object increases by 9.8 meters per second every second.
Let's look at the speed of the ball as it drops over time. This is going to help us understand how gravity causes acceleration. As you see on the screen, the ball will accelerate to a speed of 9.8 meters per second in the first second of travel. Over the next second, the speed of the ball will again increase by 9.8 meters per second, meaning it's traveling at 19.6 meters per second. The same thing will happen during the third second of time, so the ball will be traveling at 29.4 meters per second. With each second, the ball's speed increases by 9.8 meters per second.
The acceleration of gravity is so important that it has its own symbol. It is often abbreviated with the letterg. g = 9.8 m/sec^2 - that's the acceleration of gravity here on Earth for free-falling objects.
Gravity And Weight
Well, what about weight? Weight is a measure of the force of gravity acting on an object. According to Newton's laws of motion, force is directly proportional to both mass and acceleration, and the equation for force is F = m * a, where m = mass and a = acceleration. We can use this equation to solve for weight. All objects on Earth, whether they are falling, thrown, or even sitting still, experience the effect of gravity. Therefore, we can determine the weight of an object using the acceleration of gravity.
Calculation Of Weight
Let's look at an example. How much does a 100-kg man weigh on Earth?
Let's first recall the formula for force.
F = m * a
Now substitute weight for force and the acceleration of gravity (g) for acceleration.
Weight = m * g
Information copied from http://education-portal.com/academy/lesson/newtons-laws-and-weight-mass-gravity.html#lesson Links to an external site. on December 9, 2014
Suggested Objective c: Differentiate between pneumatics and hydraulic devices and functions
Hydraulics vs Pneumatics
There are almost no significant differences between hydraulics and and for non-engineers but if you examine further, there are lots of technical uniqueness in each system.
By definition alone, hydraulics is very different from pneumatics because it is used in controlling, transmitting and harnessing power using pressured fluids. The latter is dealing more on studying the impact of pressurized gases and how it influences mechanical movement. Hydraulics is frequently used in the concepts of dams, rivers, turbines and even erosion whereas pneumatics is applied in various fields of dentistry, mining and general construction among others.
The material or substance used differs between the two. In hydraulics, the substance used is an incompressible fluid medium wherein the most common example is oil. Pneumatics, on the contrary, makes use of a very compressible gas like air itself or an appropriate pure gas Links to an external site..
Another difference between the two when applied is the strength of the pressures used in their applications. Hydraulic systems use a greater amount of pressure compared to pneumatic applications. In pneumatics, only 80-100 psi (pounds per square inch) of pressure is used for its industrial applications. Hydraulic-based applications frequently use pressures that range from 1,000-5,000 psi. Nevertheless, other more advanced hydraulic systems even use pressures of up to 10,000 psi. Because of this high power demand, hydraulic systems chiefly use bigger components while pneumatic systems use smaller ones in most applications.
With regard to the control of applications, pneumatic systems are deemed to be simpler and easier to handle than hydraulic systems. Most operators say that using pneumatics is just like the light switch that makes you choose between two simple choices of ‘on’ or ‘off.’ This is true because most pneumatics is designed with simple cylinders and standard components only. An exception in the simplicity of either a hydraulic or pneumatic device would come if the entire system is automated.
Information copied from http://www.differencebetween.net/science/difference-between-hydraulics-and-pneumatics/ Links to an external site.on December 9, 2014
Advantages of Pneumatics over Hydraulics
Like hydraulics, pneumatics is a type of fluid power application where instead of an incompressible liquid, pneumatics employ gas in their system. Hydraulics present certain advantages over pneumatics, but in a given application, pneumatic powered equipment is more suitable, particularly in industries where the factory units are plumbed for compressed air.
The air used in pneumatic devices is dried and free from moisture so that it does not create any problem to the internal parts of the system. Moreover, to avoid corrosive actions, oil or lubricants are added so that friction effects can be reduced. Compressed air is used in most of the machines and in some cases compressed carbon dioxide is used. As most of the pneumatic devices are air based, they have a less complicated design and can be made of inexpensive material. Mass production techniques can be adopted to produce pneumatic systems, which not only save money but save time too.
Other major advantages are listed below.
- Initial cost is less; hydraulics equipment cost as much as twice the price of pneumatic equipment.
- A pneumatic water treatment automation system reduces the costs of installation and operation compared with conventional electrical installations. For opening and closing of underwater valves, pneumatic systems work well because they can sustain overload pressure conditions.
- Pneumatic actuators also have long life and perform well with negligible maintenance requirement throughout their life cycle.
- Very suitable for power transmission when distance of transmission is more.
The major disadvantage of pneumatic systems is that they cannot be employed for tasks that require working under high pressures. However, modern technology is working on finding better solutions![]() to this address this problem so that heavy engineering tasks can be executed using pneumatic devices. In a nutshell, in order to execute low scale engineering and mechanical tasks, pneumatic devices would be the best suited and a viable alternative over hydraulic systems.
to this address this problem so that heavy engineering tasks can be executed using pneumatic devices. In a nutshell, in order to execute low scale engineering and mechanical tasks, pneumatic devices would be the best suited and a viable alternative over hydraulic systems.
Information copied from http://www.brighthubengineering.com/fluid-mechanics-hydraulics/19006-advantages-of-pneumatics-over-hydraulics/ Links to an external site. on December 9, 2014
Hydraulic and Pneumatic Part 1
Links to an external site.
Suggested Objective d: Identify pneumatic hardware components (i.e. nail guns, vacuum tubes, air wrenches)
What are pneumatic tools?
- Pneumatic tools are powered by compressed air. Common types of these air-powered hand tools that are used in industry include buffers, nailing and stapling guns, grinders, drills, jack hammers, chipping hammers, riveting guns, sanders and wrenches.
How do you use pneumatic tools safely?
- Review the manufacturer's instruction before using a tool.
- Wear safety glasses or goggles, or a face shield (with safety glasses or goggles), and, where necessary, safety shoes or boots and hearing protection.
- Post warning signs where pneumatic tools are used. Set up screens or shields in areas where nearby workers may be exposed to flying fragments, chips, dust, and excessive noise.
- Ensure that the compressed air supplied to the tool is clean and dry. Dust, moisture, and corrosive fumes can damage a tool. An in-line regulator filter and lubricator increases tool life.
- Keep tools clean and lubricated, and maintain them according to the manufacturers' instructions.
- Use only the attachments that the manufacturer recommends for the tools you are using.
- Be careful to prevent hands, feet, or body from injury in case the machine slips or the tool breaks.
- Reduce physical fatigue by supporting heavy tools with a counter-balance wherever possible.
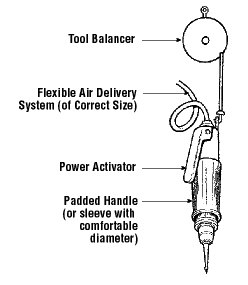
How should you handle air hoses?
- Use the proper hose and fittings of the correct diameter.
- Use hoses specifically designed to resist abrasion, cutting, crushing and failure from continuous flexing.
- Choose air-supply hoses that have a minimum working pressure rating of 1035 kPa (150 psig) or 150% of the maximum pressure produced in the system, whichever is higher.
- Check hoses regularly for cuts, bulges and abrasions. Tag and replace, if defective.
- Blow out the air line before connecting a tool. Hold hose firmly and blow away from yourself and others.
- Make sure that hose connections fit properly and are equipped with a mechanical means of securing the connection (e.g., chain, wire, or positive locking device).
- Install quick disconnects of a pressure-release type rather than a disengagement type. Attach the male end of the connector to the tool, NOT the hose.
- Do not operate the tool at a pressure above the manufacturer's rating.
- Turn off the air pressure to hose when not in use or when changing power tools.
- Do not carry a pneumatic tool by its hose.
- Avoid creating trip hazards caused by hoses laid across walkways or curled underfoot.
- Do not use compressed air to blow debris or to clean dirt from clothes.

What should you avoid with a compressed air?
- Cleaning with compressed air is dangerous.
- Do not use compressed air for cleaning unless no alternate method of cleaning is available. The nozzle pressure MUST remain below 207 kPa (30 psi). Personal protective equipment and effective chip guarding techniques must be used.
- Two acceptable methods of meeting the "below 207 kPa (30 psi)" requirement are illustrated below.


Information copied from http://www.ccohs.ca/oshanswers/safety_haz/power_tools/pneumat.html
Links to an external site.on December 9, 2014
Air Compressors and Pneumatic Tools
Links to an external site.
Suggested Objective e: Investigate atmospheric and vacuum pressure
Atmospheric Pressure
The surface of the earth is at the bottom of an atmospheric sea. The standard atmospheric pressure is measured in various units:
1 atmosphere = 760 mmHg = 29.92 inHg = 14.7 lb/in2 = 101.3 KPaThe fundamental SI unit of pressure Links to an external site. is the Pascal (Pa), but it is a small unit so kPa is the most common direct pressure unit for atmospheric pressure. Since the static fluid pressure Links to an external site. is dependent only upon density and depth, choosing a liquid of standard density like mercury or water allows you to express the pressure in units of height or depth, e.g., mmHg or inches of water. The mercury barometer Links to an external site. is the standard instrument for atmospheric pressure measurement in weather reporting. The decrease in atmospheric pressure with height can be predicted from thebarometric formula Links to an external site..
The unit mmHg is often called torr, particularly in vacuum applications: 760 mmHg = 760 torr
For weather applications, the standard atmospheric pressure is often called 1 bar or 1000 millibars. This has been found to be convenient for recording the relatively small deviations from standard atmospheric pressure with normal weather patterns.
Information copied from http://hyperphysics.phy-astr.gsu.edu/hbase/pman.html Links to an external site.on December 9, 2014
he pressure in a fluid is defined as
"the normal force per unit area exerted on a imaginary or real plane surface in a fluid or a gas"
The equation for pressure can expressed as:
p = F / A (1)
where
p = pressure (lb/in2 (psi) or lb/ft2 (psf), N/m2 or kg/ms2 (Pa))
F = force (1), N)
A = area (in2 or ft2, m2)
1) In the English Engineering System Links to an external site. special care must be taken for the force unit. The basic unit for mass is the pound mass (lbm) and the unit for the force is the pound (lb) or pound force (lbf).
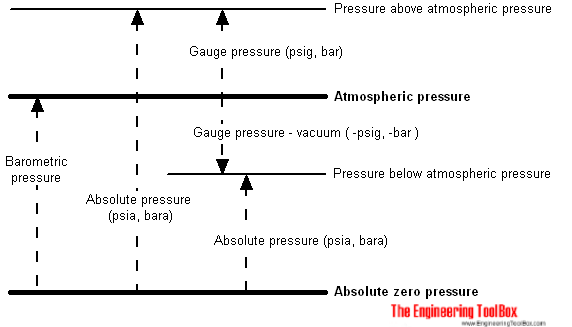
Absolute Pressure
The absolute pressure - pabs - is measured relative to the absolute zero pressure - the pressure that would occur at absolute vacuum. All calculation involving the gas laws requires pressure (and temperature) to be in absolute units.
Gauge Pressure
A gauge is often used to measure the pressure difference between a system and the surrounding atmosphere. This pressure is often called the gauge pressure and can be expressed as
pg = ps - patm (2)
where
pg = gauge pressure
ps = system pressure
patm = atmospheric pressure
Atmospheric Pressure
Atmospheric pressure is pressure in the surrounding air at - or "close" to - the surface of the earth. The atmospheric pressure vary with temperature and altitude above sea level.
Standard Atmospheric Pressure
Standard Atmospheric Pressure (atm) is used as a reference for gas densities and volumes. The Standard Atmospheric Pressure is defined at sea-level at 273oK (0oC) and is 1.01325 bar or 101325 Pa (absolute). The temperature of 293oK (20oC) is also used.
In imperial units the Standard Atmospheric Pressure is 14.696 psi.
- 1 atm = 1.01325 bar = 101.3 kPa = 14.696 psi (lbf/in2)= 760 mmHg =10.33 mH2O = 760 torr = 29.92 inHg = 1013 mbar = 1.0332 kgf/cm2 = 33.90 ftH2O
Pressure Units
Since 1 Pa is a small pressure unit, the unit hectoPascal (hPa) is widely used, especially in meteorology. The unit kiloPascal (kPa) is commonly used design of technical applications like HVAC systems, piping systems and similar.
- 1 hectoPascal = 100 Pascal = 1 millibar
- 1 kiloPascal = 1000 Pascal
Some Pressure Levels
- 10 Pa - the pressure below 1 mm of water
- 1 kPa - approximately the pressure exerted by a 10 g of mass on a 1 cm2 area
- 10 kPa - the pressure below 1 m of water, or the drop in air pressure when moving from sea level to 1000 m elevation
- 10 MPa - nozzle pressure in a "high pressure" washer
- 10 GPa - pressure enough to form diamonds
Some Alternative Units of Pressure
- 1 bar - 100,000 Pa
- 1 millibar - 100 Pa
- 1 atmosphere - 101,325 Pa
- 1 mm Hg - 133 Pa
- 1 inch Hg - 3,386 Pa
A torr (often used in vacuum applications) is named after Torricelli and is the pressure produced by a column of mercury 1 mm high - equals to 1 / 760th of an atmosphere.
- 1 atm = 760 torr = 14.696 psi
Pounds per square inch (psi) was common in U.K. but has now been replaced in almost every country except in the U.S. by the SI units. Since atmospheric pressure is 14.696 psi - a column of air on a area of one square inch area from the Earth's surface to the space - weights 14.696 pounds.
The bar (bar) is common in the industry. One bar is 100,000 Pa, and for most practical purposes can be approximated to one atmosphere even if
1 Bar = 0.9869 atm
There are 1,000 millibar (mbar) in one bar, a unit common in meteorology and weather applications.
1 millibar = 0.001 bar = 0.750 torr = 100 Pa
Information copied from http://www.engineeringtoolbox.com/pressure-d_587.html Links to an external site.on December 9, 2014 - you can perform practice calculations from this site.
Basics of Vacuum
Evacuating air from a closed volume develops a pressure differential between the volume and the surrounding atmosphere. If this closed volume is bound by the surface of a vacuum cup and a workpiece, atmospheric pressure will press the two objects together. The amount of holding force depends on the surface area shared by the two objects and the vacuum level. In an industrial vacuum system, a vacuum pump Links to an external site. or generator removes air from a system to create a pressure differential.
Because it is virtually impossible to remove all the air molecules from a container, a perfect vacuum cannot be achieved. Of course, as more air is removed, the pressure differential increases, and the potential vacuum force becomes greater.
The vacuum level is determined by the pressure differential between the evacuated volume and the surrounding atmosphere. Several units of measure can be used. Most refer to the height of a column of mercury — usually inches of mercury (in.-Hg) or millimeters of mercury (mm-Hg). The common metric unit for vacuum measurement is the millibar, or mbar. Other pressure units sometimes used to express vacuum include the interrelated units of atmospheres, torr, and microns. One standard atmosphere equals 14.7 psi (29.92 in.-Hg). Any fraction of an atmosphere is a partial vacuum and equates with negative gauge pressure. A torr is defined as 1/760 of an atmosphere and can also be thought of as 1 mm-Hg, where 760 mm-Hg equals 29.92 in.-Hg. Even smaller is the micron, defined as 0.001 torr. However, these units are used most often when dealing with near-perfect vacuums, usually under laboratory conditions, and seldom in fluid power applications.
Atmospheric pressure is measured with a barometer. A barometer consists of an evacuated vertical tube with its top end closed and its bottom end resting in a container of mercury that is open to the atmosphere, Figure 1. The pressure exerted by the atmosphere acts on the exposed surface of the liquid to force mercury up into the tube. Sea level atmospheric pressure will support a mercury column generally not more than 29.92-in. high. Thus, the standard for atmospheric pressure at sea level is 29.92 in.-Hg, which translates to an absolute pressure of 14.69 psia.
The two basic reference points in all these measurements are standard atmospheric pressure and a perfect vacuum. At atmospheric pressure, the value 0 in.-Hg is equivalent to 14.7 psia. At the opposite reference point, 0 psia, — a perfect vacuum (if it could be attained) — would have a value equal to the other extreme of its range, 29.92 in.-Hg. However, calculating work forces or changes in volume in vacuum systems requires conversions to negative gauge pressure (psig) or absolute pressure (psia).
Atmospheric pressure is assigned the value of zero on the dials of most pressure gauges. Vacuum measurements must, therefore, be less than zero. Negative gage pressure generally is defined as the difference between a given system vacuum and atmospheric pressure.
Vacuum measurement
Several types of gauges measure vacuum level. A Bourdon tube-type gauge is compact and the most widely used device for monitoring vacuum system operation and performance. Measurement is based on the deformation of a curved elastic Bourdon tube when vacuum is applied to the gauge's port. With the proper linkage, compound Bourdon tube gauges indicate both vacuum and positive pressure.
An electronic counterpart to the vacuum gauge is the transducer. Vacuum or pressure deflects an elastic metal diaphragm. This deflection varies electrical characteristics of interconnected circuitry to produce an electronic signal that represents the vacuum level.
A U-tube manometer, Figure 2, indicates the difference between two pressures. In its simplest form, a manometer is a transparent U-tube half-filled with mercury. With both ends of the tube exposed to atmospheric pressure, the mercury level in each leg is the same. Applying a vacuum to one leg causes the mercury to rise in that leg and to fall in the other.
The difference in height between the two levels indicates the vacuum level. Manometers can measure vacuum directly to 29.25 in.-Hg.
An absolute pressure gauge shows the pressure above a theoretical perfect vacuum. It has the same U-shape as the manometer, but one leg of the absolute pressure gauge is sealed, Figure 3. Mercury fills this sealed leg when the gauge is at rest. Applying vacuum to the unsealed leg lowers the mercury level in the sealed leg. The vacuum level is measured with a sliding scale placed with its zero point at the mercury level in the unsealed leg. Thus, this gauge compensates for changes in atmospheric pressure.
Information copied from http://hydraulicspneumatics.com/200/TechZone/Vacuum/Article/False/6460/TechZone-Vacuum Links to an external site.on December 9, 2014
Bill Nye - Atmospheric Pressure
Links to an external site.
Suggested Objective f: Demonstrate how pressure differences are related to force
Pressure
Pressure is defined as force per unit area. It is usually more convenient to use pressure rather than force to describe the influences upon fluid behavior. The standard unit for pressure is the Pascal, which is a Newton per square meter.
For an object sitting on a surface, the force pressing on the surface is the weight Links to an external site. of the object, but in different orientations it might have a different area in contact with the surface and therefore exert a different pressure.
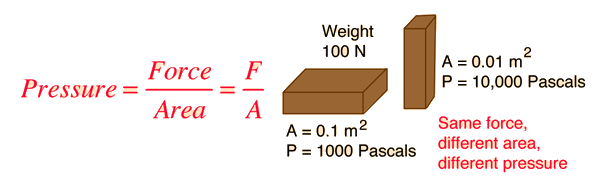
Pressure calculation. Links to an external site.
There are many physical situations where pressure is the most important variable. If you are peeling an apple, then pressure is the key variable: if the knife is sharp, then the area of contact is small and you can peel with less force exerted on the blade. If you must get an injection, then pressure is the most important variable in getting the needle through your skin: it is better to have a sharp needle than a dull one since the smaller area of contact implies that less force is required to push the needle through the skin.
When you deal with the pressure of a liquid at rest Links to an external site., the medium is treated as a continuous distribution of matter. But when you deal with a gas pressure Links to an external site., it must be approached as an average pressure from molecular collisions with the walls.
Pressure in a fluid can be seen to be a measure of energy per unit volume Links to an external site. by means of the definition of work Links to an external site.. This energy is related to other forms of fluid energy by theBernoulli equation Links to an external site..
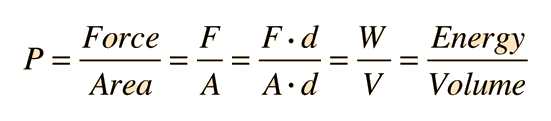
Information copied from http://hyperphysics.phy-astr.gsu.edu/hbase/press.html Links to an external site. on December 9, 2014
Pressure and force are related, and so you can calculate one if you know the other by using the physics equation, F = P/A. Because pressure is force divided by area, its meter-kilogram-second (MKS) units are newtons per square meter, or N/m2. In the foot-pound-second (FPS) system, the units are pounds per square inch, or psi.
The unit newtons per square meter is so common in physics that it has a special name: the pascal, which equals 1 newton per square meter. The pascal is abbreviated as Pa.
You don’t have to be underwater to experience pressure from a fluid. Air exerts pressure, too, due to the weight of the air above you. Here’s how much pressure the air exerts on you at sea level:
![]()
The air pressure at sea level is a standard pressure that people refer to as 1 atmosphere(abbreviated atm):
![]()
If you convert an atmosphere to pounds per square inch, it’s about 14.7 psi. That means that 14.7 pounds of force are pressing in on every square inch of your body at sea level.
Your body pushes back with 14.7 psi, so you don’t feel any pressure on you at all. But if you suddenly got transported to outer space, the inward pressure of the air pushing on you would be gone, and all that would remain would be the 14.7 pounds per square inch your body exerted outward. You wouldn’t explode, but your lungs could burst if you tried to hold your breath. The change in pressure could also cause the nitrogen in your blood to form bubbles and give you the bends!
Here’s a pressure example problem using water pressure. Say you’re in your neighbor’s pool, waiting near the bottom until your neighbors give up trying to chase you off and go back into the house. You’re near the deep end of the pool, and using the handy pressure gauge you always carry, you measure the pressure on the back of your hand as
![]()
What force does the water exert on the back of your hand? The back of your hand has an area of about
![]()
You reason that if P = F/A, then the following is true:
F = PA
Plugging in the numbers and solving gives you the answer:

Yikes. A thousand newtons! You whip out your underwater calculator to find that’s about 230 pounds. Forces add up quickly when you’re underwater because water is a heavy liquid. The force you feel is the weight of the water above you.
Information copied from http://www.dummies.com/how-to/content/how-to-calculate-force-based-on-pressure.html Links to an external site.on December 9, 2014
Force and Pressure
Links to an external site.
Pressure versus Force
Links to an external site.







