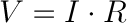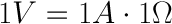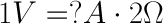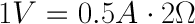Unit 10 Competency 2 - Examine principles of magnetism, electric fields, and electricity
Suggested Objective a: Analyze and explain the relationship between electricity and magnetism (Characteristics of static charge and how a static charge is generated; Electric field, electric potential, current, voltage, and resistance as related to Ohm’s Law.)
 Magnetic Field Basics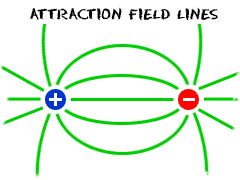 Magnetic fields are different from electric fields. Although both types of fields are interconnected, they do different things. The idea of magnetic field lines and magnetic fields was first examined by Michael Faraday and later by James Clerk Maxwell. Both of these English scientists made great discoveries in the field of electromagnetism. Magnetic fields are areas where an object exhibits a magnetic influence. The fields affect neighboring objects along things called magnetic field lines. A magnetic object can attract or push away another magnetic object. You also need to remember that magnetic forces are NOT related to gravity Links to an external site.. The amount of gravity is based on an object's mass, while magnetic strength is based on the material that the object is made of. 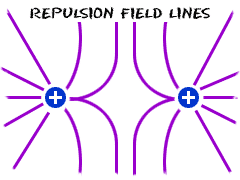 If you place an object in a magnetic field, it will be affected, and the effect will happen along field lines. Many classroom experiments watch small pieces of iron(Fe) line up around magnets along the field lines. Magnetic poles are the points where the magnetic field lines begin and end. Field lines converge or come together at the poles. You have probably heard of the poles of the Earth. Those poles are places where our planets field lines come together. We call those poles north and south because that's where they're located on Earth. All magnetic objects have field lines and poles. It can be as small as an atom or as large as a star.
|
Information copied from http://www.physics4kids.com/files/elec_magneticfield.html Links to an external site.on December 9, 2014
It turns out that electricity and magnetism are two aspects of the same force. The relation was first stated by the Scottish physicist, James Maxwell.
Ways to Demonstrate the Relation:
- Every time an electric charge moves, a magnetic field is created, and every time a magnetic field is varied an electric field is created.
- If you have a coil of wire around a core of metal and pass a current through the coil, a magnetic field is generated. This is called an electromagnet. Moving electric charges generates magnetic fields.
- In the same way, if you move a wire circuit through a magnetic field, it will generate an electric current. Varying magnetic fields will generate an electric current.
Information copied from http://faculty.stedwards.edu/davidw/scie/physics/relation.html Links to an external site. on December 9, 2014
Electricity and Magnetism
Links to an external site.
The Wonders of Electricity and Magnetism Links to an external site.
What is Static Electricity?
You walk across the rug, reach for the doorknob and..........ZAP!!! You get a static shock.
Or, you come inside from the cold, pull off your hat and......static hair!!
The static electricity makes your hair stand straight out from your head.
What is going on here? And why is static more of a problem in the winter?
Everything Is Made Of Atoms
Imagine a pure gold ring. Divide it in half and give one of the halves away. Keep dividing and dividing and dividing. Soon you will have a piece so small you will not be able to see it without a microscope. It may be very, very small, but it is still a piece of gold.
If you could keep dividing it into smaller and smaller pieces, you would finally get to the smallest piece of gold possible. It is called an atom. If you divided it into smaller pieces, it would no longer be gold.
Everything around us is made of atoms and scientists so far know of 118 different kinds. These different kinds of atoms are called "elements." There are 98 elements that exist naturally (although some are only found in very small amounts). Four of these 118 elements have reportedly been discovered, but have not yet been confirmed.
Atoms join together in many different combinations to form molecules, and create all of the materials you see around you.
Parts Of An Atom
So what are atoms made of? In the middle of each atom is a "nucleus." The nucleus contains two kinds of tiny particles, called protons and neutrons. Orbiting around the nucleus are even smaller particles called electrons. The 115 kinds of atoms are different from each other because they have different numbers of protons, neutrons and electrons.
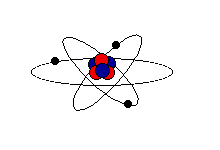
It is useful to think of a model of the atom as similar to the solar system. The nucleus is in the center of the atom, like the sun in the center of the solar system. The electrons orbit around the nucleus like the planets around the sun.
Just like in the solar system, the nucleus is large compared to the electrons. The atom is mostly empty space. And the electrons are very far away from the nucleus. While this model is not completely accurate, we can use it to help us understand static electricity.
(Note: A more accurate model would show the electrons moving in 3- dimensional volumes with different shapes, called orbitals. This may be discussed in a future issue.)
Protons, neutrons and electrons are very different from each other. They have their own properties, or characteristics. One of these properties is called an electrical charge. Protons have what we call a "positive" (+) charge. Electrons have a "negative" (-) charge. Neutrons have no charge, they are neutral.
The charge of one proton is equal in strength to the charge of one electron. When the number of protons in an atom equals the number of electrons, the atom itself has no overall charge, it is neutral.
Electrons Can Move
The protons and neutrons in the nucleus are held together very tightly. Normally the nucleus does not change. But some of the outer electrons are held very loosely. They can move from one atom to another.
An atom that loses electrons has more positive charges (protons) than negative charges (electrons). It is positively charged. An atom that gains electrons has more negative than positive particles. It has a negative charge. A charged atom is called an "ion."
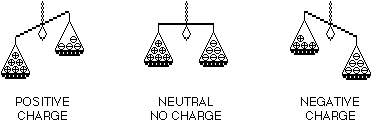
Some materials hold their electrons very tightly. Electrons do not move through them very well. These things are called insulators. Plastic, cloth, glass and dry air are good insulators. Other materials have some loosely held electrons, which move through them very easily. These are called conductors. Most metals are good conductors.
How can we move electrons from one place to another? One very common way is to rub two objects together. If they are made of different materials, and are both insulators, electrons may be transferred (or moved) from one to the other. The more rubbing, the more electrons move, and the larger the static charge that builds up. (Scientists believe that it is not the rubbing or friction that causes electrons to move. It is simply the contact between two different materials. Rubbing just increases the contact area between them.)
Static electricity is the imbalance of
positive and negative charges.
Opposites Attract
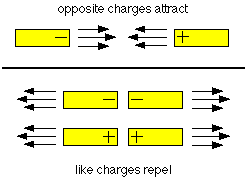Now, positive and negative charges behave in interesting ways. Did you ever hear the saying that opposites attract? Well, it's true. Two things with opposite, or different charges (a positive and a negative) will attract, or pull towards each other. Things with the same charge (two positives or two negatives) will repel, or push away from each other.
A charged object will also attract something that is neutral. Think about how you can make a balloon stick to the wall.
If you charge a balloon by rubbing it on your hair, it picks up extra electrons and has a negative charge. Holding it near a neutral object will make the charges in that object move.
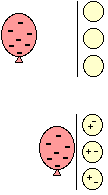If it is a conductor, many electrons move easily to the other side, as far from the balloon as possible.
If it is an insulator, the electrons in the atoms and molecules can only move very slightly to one side, away from the balloon.
In either case, there are more positive charges closer to the negative balloon.
Opposites attract. The balloon sticks. (At least until the electrons on the balloon slowly leak off.) It works the same way for neutral and positively charged objects.
So what does all this have to do with static shocks? Or static electricity in hair?
When you take off your wool hat, it rubs against your hair. Electrons move from your hair to the hat. A static charge builds up and now each of the hairs has the same positive charge.
Remember, things with the same charge repel each other. So the hairs try to get as far from each other as possible. The farthest they can get is by standing up and away from the others. And that is how static electricity causes a bad hair day!
Information copied from http://www.sciencemadesimple.com/static.html Links to an external site.on December 9, 2014
Electricity Basics
When beginning to explore the world of electricity and electronics, it is vital to start by understanding the basics of voltage, current, and resistance. These are the three basic building blocks required to manipulate and utilize electricity. At first, these concepts can be difficult to understand because we cannot “see” them. One cannot see with the naked eye the energy flowing through a wire or the voltage of a battery sitting on a table. Even the lightning in the sky, while visible, is not truly the energy exchange happening from the clouds to the earth, but a reaction in the air to the energy passing through it. In order to detect this energy transfer, we must use measurement tools such as multimeters, spectrum analyzers, and oscilloscopes to visualize what is happening with the charge in a system. Fear not, however, this tutorial will give you the basic understanding of voltage, current, and resistance and how the three relate to each other.
Electrical Charge
Electricity is the movement of electrons. Electrons create charge, which we can harness to do work. Your lightbulb, your stereo, your phone, etc., are all harnessing the movement of the electrons in order to do work. They all operate using the same basic power source: the movement of electrons.
The three basic principles for this tutorial can be explained using electrons, or more specifically, the charge they create:
- Voltage is the difference in charge between two points.
- Current is the rate at which charge is flowing.
- Resistance is a material’s tendency to resist the flow of charge (current).
So, when we talk about these values, we’re really describing the movement of charge, and thus, the behavior of electrons. A circuit is a closed loop that allows charge to move from one place to another. Components in the circuit allow us to control this charge and use it to do work.
Georg Ohm Links to an external site. was a Bavarian scientist who studied electricity. Ohm starts by describing a unit of resistance that is defined by current and voltage. So, let’s start with voltage and go from there.
Voltage
We define voltage as the amount of potential energy between two points on a circuit. One point has more charge than another. This difference in charge between the two points is called voltage. It is measured in volts, which, technically, is the potential energy difference between two points that will impart one joule of energy per coulomb of charge that passes through it (don’t panic if this makes no sense, all will be explained). The unit “volt” is named after the Italian physicist Alessandro Volta Links to an external site. who invented what is considered the first chemical battery. Voltage is represented in equations and schematics by the letter “V”.
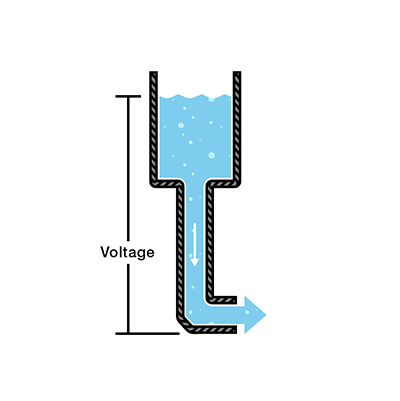
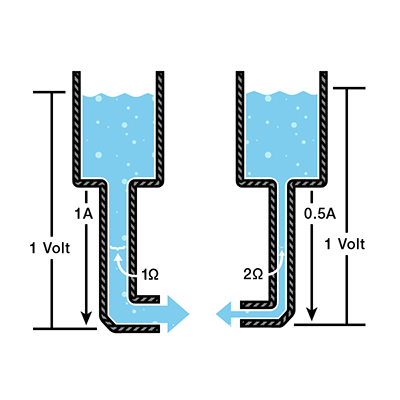
When describing voltage, current, and resistance, a common analogy is a water tank. In this analogy, charge is represented by the water amount, voltage is represented by the water pressure, and current is represented by the water flow. So for this analogy, remember:
- Water = Charge
- Pressure = Voltage
- Flow = Current
Consider a water tank at a certain height above the ground. At the bottom of this tank there is a hose.
The pressure at the end of the hose can represent voltage. The water in the tank represents charge. The more water in the tank, the higher the charge, the more pressure is measured at the end of the hose.
We can think of this tank as a battery, a place where we store a certain amount of energy and then release it. If we drain our tank a certain amount, the pressure created at the end of the hose goes down. We can think of this as decreasing voltage, like when a flashlight gets dimmer as the batteries run down. There is also a decrease in the amount of water that will flow through the hose. Less pressure means less water is flowing, which brings us to current.
Current
We can think of the amount of water flowing through the hose from the tank as current. The higher the pressure, the higher the flow, and vice-versa. With water, we would measure the volume of the water flowing through the hose over a certain period of time. With electricity, we measure the amount of charge flowing through the circuit over a period of time. Current is measured in Amperes (usually just referred to as “Amps”). An ampere is defined as 6.241*1018electrons (1 Coulomb) per second passing through a point in a circuit. Amps are represented in equations by the letter “I”.
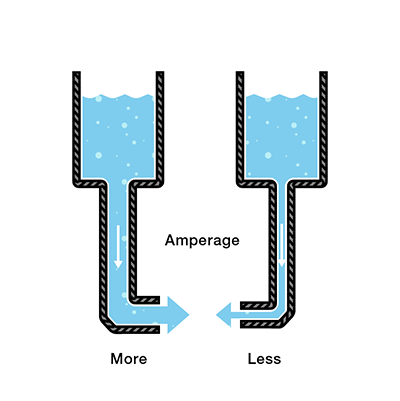
Let’s say now that we have two tanks, each with a hose coming from the bottom. Each tank has the exact same amount of water, but the hose on one tank is narrower than the hose on the other.
We measure the same amount of pressure at the end of either hose, but when the water begins to flow, the flow rate of the water in the tank with the narrower hose will be less than the flow rate of the water in the tank with the wider hose. In electrical terms, the current through the narrower hose is less than the current through the wider hose. If we want the flow to be the same through both hoses, we have to increase the amount of water (charge) in the tank with the narrower hose.
This increases the pressure (voltage) at the end of the narrower hose, pushing more water through the tank. This is analogous to an increase in voltage that causes an increase in current.
Now we’re starting to see the relationship between voltage and current. But there is a third factor to be considered here: the width of the hose. In this analogy, the width of the hose is the resistance. This means we need to add another term to our model:
- Water = Charge (measured in Coulombs)
- Pressure = Voltage (measured in Volts)
- Flow = Current (measured in Amperes, or “Amps” for short)
- Hose Width = Resistance
Resistance
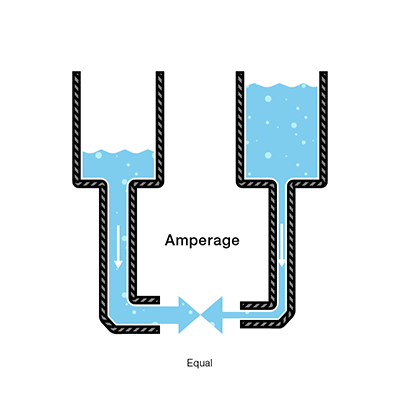
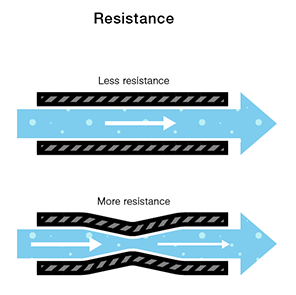
Consider again our two water tanks, one with a narrow pipe and one with a wide pipe.
It stands to reason that we can’t fit as much volume through a narrow pipe than a wider one at the same pressure. This is resistance. The narrow pipe “resists” the flow of water through it even though the water is at the same pressure as the tank with the wider pipe.
In electrical terms, this is represented by two circuits with equal voltages and different resistances. The circuit with the higher resistance will allow less charge to flow, meaning the circuit with higher resistance has less current flowing through it.
This brings us back to Georg Ohm. Ohm defines the unit of resistance of “1 Ohm” as the resistance between two points in a conductor where the application of 1 volt will push 1 ampere, or 6.241×1018 electrons. This value is usually represented in schematics with the greek letter “Ω”, which is called omega, and pronounced “ohm”.
Ohm's Law
Combining the elements of voltage, current, and resistance, Ohm developed the formula:
Where
- V = Voltage in volts
- I = Current in amps
- R = Resistance in ohms
This is called Ohm’s law. Let’s say, for example, that we have a circuit with the potential of 1 volt, a current of 1 amp, and resistance of 1 ohm. Using Ohm’s Law we can say:
Let’s say this represents our tank with a wide hose. The amount of water in the tank is defined as 1 volt and the “narrowness” (resistance to flow) of the hose is defined as 1 ohm. Using Ohms Law, this gives us a flow (current) of 1 amp.
Using this analogy, let’s now look at the tank with the narrow hose. Because the hose is narrower, its resistance to flow is higher. Let’s define this resistance as 2 ohms. The amount of water in the tank is the same as the other tank, so, using Ohm’s Law, our equation for the tank with the narrow hose is
But what is the current? Because the resistance is greater, and the voltage is the same, this gives us a current value of 0.5 amps:
So, the current is lower in the tank with higher resistance. Now we can see that if we know two of the values for Ohm’s law, we can solve for the third. Let’s demonstrate this with an experiment.
An Ohm's Law Experiment
For this experiment, we want to use a 9 volt battery to power an LED. LEDs are fragile and can only have a certain amount of current flowing through them before they burn out. In the documentation for an LED, there will always be a “current rating”. This is the maximum amount of current that can flow through the particular LED before it burns out.
Information copied from https://learn.sparkfun.com/tutorials/voltage-current-resistance-and-ohms-law Links to an external site. on December 9, 2014
What Is Current and Voltage in a Circuit?
Links to an external site.
What Is Ohm's Law?
Links to an external site.
Suggested Objective b: Calculate resistance with voltage and current
Formulas: V = I × R I = V / R R = V / I
The mathematical formulas of Ohm's Law
Ohm's Law can be rewritten in three ways for calculating current, resistance, and voltage.
If a current I should flow through a resistor R, the voltage V can be calculated.
First Version of the (voltage) formula: V = I × R
If there is a voltage V across a resistor R, a current I flows through it. I can be calculated.
Second Version of the (current) formula: I = V / R
If a current I flows through a resistor, and there is a voltage V across the resistor. R can be calculated.
Third Version of the (resistance) formula: R = V / I
All of these variations of the so called "Ohm's Law" are mathematically equal to one another.
| Name | Formula sign | Unit | Symbol |
| voltage | V or E | volt | V |
| current | I | ampere (amp) | A |
| resistance | R | ohm | Ω |
| power | P | watt | W |
|
What is the formula for electrical current? When the current is constant: I = Δ Q / Δ t I is the current in amps (A) Δ Q is the electric charge in coulombs (C), that flows at time duration of Δ t in seconds (s). Voltage V = current I × resistance R Power P = voltage V × current I |
In electrical conductors, in which the current and voltage are proportional
to each other, ohm's law apply: V ~ I or V ⁄ I = const.
Constantan wires or other metal wires held at a constant temperature meet well ohm's law.
"V ⁄ I = R = const." ist not the law of ohm. It is the definition of the resistance.
Thereafter, in every point, even with a bent curve, the resistance value can be calculated.
For many electrical components such as diodes ohm's law does not apply.
"Ohm's Law" has not been invented by Mr. Ohm
|
"U ⁄ I = R = const." is not the law of Ohm or Ohm's law. It is the definition of the resistance. |
 |
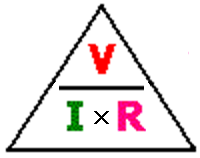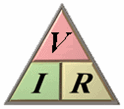
Tip: Ohm's magic triangle The magic V I R triangle can be used to calculate all formulations of ohm's law. Use a finger to hide the value to be calculated. The other two values then show how to do the calculation. |
The symbol I or J = Latin: influare, international ampere, and R = resistance. V = voltage or
electric potential difference, also called voltage drop, or E = electromotive force (emf = voltage).
|
Voltage drop calculations - DC / single phase calculation The voltage drop V in volts (V) is equal to the wire current I in amps (A) times twice the wire length L in feet (ft) times the wire resistance per 1000 feet R in ohms (Ω / kft) divided by 1000: Vdrop (V) = Iwire (A) × Rwire (Ω) = Iwire (A) × (2 × L (ft) × Rwire (Ω / kft) / 1000 (ft / kft)) The voltage drop V in volts (V) is equal to the wire current I in amps (A) times twice the wire length L in meters (m) times the wire resistance per 1000 meters R in ohms (Ω / km) divided by 1000: Vdrop (V) = Iwire (A) × Rwire (Ω) = Iwire (A) × (2 × L (m) × Rwire (Ω / km) / 1000 (m / km)) |
If the unit of power P = I × V and of voltage V = I · R is needed,
look for 
"The Big Power Formulas":
Calculations: power (watt), voltage, current, resistance
Links to an external site.
Some persons think that Georg Simon Ohm calculated the "specific resistance".
Therefore they think that only the following can be the true ohm's law.
|
Electrical conductivity (conductance) σ (sigma) = 1/ρ
Specific electrical resistance (resistivity) ρ (rho) = 1/σ
| Electrical
Links to an external site. conductor Links to an external site. |
Electrical conductivity
Links to an external site. Electrical conductance Links to an external site. |
Electrical resistivity
Links to an external site. Specific resistance Links to an external site. |
| silver | σ = 62 S·m/mm² | ρ = 0.0161 Ohm∙mm²/m |
| copper | σ = 58 S·m/mm² | ρ = 0.0172 Ohm∙mm²/m |
| gold | σ = 41 S·m/mm² | ρ = 0.0244 Ohm∙mm²/m |
| aluminium | σ = 36 S·m/mm² | ρ = 0.0277 Ohm∙mm²/m |
| constantan | σ = 2.0 S·m/mm² | ρ = 0.5000 Ohm∙mm²/m |
Difference between electrical resistivity and electrical conductivity
| The conductance in siemens is the reciprocal of the resistance in ohms. |
|
Simply enter the value to the left or the right side. The calculator works in both directions of the ↔ sign. |
|
Electrical conductivity σ S · m / mm² |
↔ |
Specific elec. resistance ρ Ohm ∙ mm² / m |
|
| σ = 1 / ρ | ρ = 1 / σ | ||
| siemens S = 1/Ω or ohm Ω = 1/S | |||
|
The value of the electrical conductivity (conductance) and the specific electrical resistance (resistivity) is a temperature dependent material constant. Mostly it is given at 20 or 25°C. Resistance R = ρ × (l / A) or R = l / (σ × A) |
|
For all conductors the specific resistivity changes with the temperature. In a limited temperature range it is approximately linear:  where α is the temperature coefficient, T is the temperature and T0 is any temperature,such as T0 = 293.15 K = 20°C at which the electrical resistivity ρ (T0) is known. |
Cross-sectional area - cross section - slice plane
Now there is the question:
How can we calculate the cross sectional area (slice plane) A
from the wire diameter d and vice versa?
Calculation of the cross section A (slice plane) from diameter d:
r = radius of the wire
d = diameter of the wire
Calculation diameter d from cross section A (slice plane):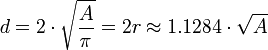
Cross section A of the wire in mm2 inserted in this formula gives the diameter d in mm.
Calculation − Round cables and wires:
• Diameter to cross section and vice versa •
Links to an external site.
Electric voltage V = I × R (Ohm's law VIR)
Electrical voltage = amperage × resistance (Ohm's law)
Please enter two values, the third value will be calculated.
|
|||||||||||||||
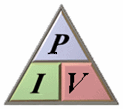
Electric power P = I × V (Power law PIV)
Electric power = amperage × voltage (Watt's Law)
Please enter two values, the third value will be calculated.
|
|||||||||||||||
| Ohm's law. V = I × R, where V is the potential across a circuit element, I is the current through it, and R is its resistance. This is not a generally applicable definition of resistance. It is only applicable to ohmic resistors, those whose resistance R is constant over the range of interest and V obeys a strictly linear relation to I. Materials are said to be ohmic when V depends linearly on R. Metals are ohmic so long as one holds their temperature constant. But changing the temperature of a metal changes R slightly. When the current changes rapidly, as when turning on a light, or when using AC sources, slightly non-linear and non-ohmic behavior can be observed. For non-ohmic resistors, R is current-dependent and the definition R = dV/dI is far more useful. This is sometimes called the dynamic resistance. Solid state devices such as thermistors are non-ohmic and non-linear. A thermistor's resistance decreases as it warms up, so its dynamic resistance is negative. Tunnel diodes and some electrochemical processes have a complicated I to V curve with a negative resistance region of operation. The dependence of resistance on current is partly due to the change in the device's temperature with increasing current, but other subtle processes also contribute to change in resistance in solid state devices. |
Information copied from http://www.sengpielaudio.com/calculator-ohmslaw.htm Links to an external site. on December 9, 2014
The above site has calculators for you to use to learn how to calculate resistance. Try various examples. Take careful notes!
Suggested Objective c: Evaluate schematic diagrams to analyze the current flow in series and parallel electric circuits, given the component resistances and the imposed electric potential
Two or more electrical devices in a circuit can be connected by series connections or by parallel connections. When all the devices are connected using series connections, the circuit is referred to
as a series circuit. In a series circuit, each device is connected in a manner such that there is only one pathway by which charge can traverse the external circuit. Each charge passing through the loop of the external circuit will pass through each resistor in consecutive fashion.
A short comparison and contrast between series and parallel circuits was made in the previous section of Lesson 4 Links to an external site.. In that section, it was emphasized that the act of adding more resistors to a series circuit results in the rather expected result of having more overall resistance. Since there is only one pathway through the circuit, every charge encounters the resistance of every device; so adding more devices results in more overall resistance. This increased resistance serves to reduce the rate at which charge flows (also known as the current Links to an external site.).
Equivalent Resistance and Current
Charge flows together through the external circuit at a rate that is everywhere the same. The current is no greater at one location as it is at another location. The actual amount of current varies inversely with the amount of overall resistance. There is a clear relationship between the resistance of the individual resistors and the overall resistance of the collection of resistors. As far as the battery that is pumping the charge is concerned, the presence of two 6-Ω ;resistors in series would be equivalent to having one 12-Ω resistor in the circuit. The presence of three 6-Ω resistors in series would be equivalent to having one 18-Ω resistor in the circuit. And the presence of four 6-Ω resistors in series would be equivalent to having one 24-Ω resistor in the circuit.
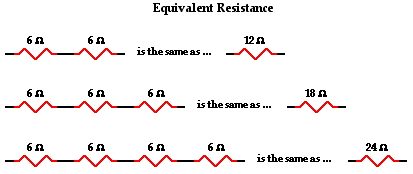
This is the concept of equivalent resistance. The equivalent resistance of a circuit is the amount of resistance that a single resistor would need in order to equal the overall effect of the collection of resistors that are present in the circuit. For series circuits, the mathematical formula for computing the equivalent resistance (Req) is
Req = R1 + R2 + R3 + ...
where R1, R2, and R3 are the resistance values of the individual resistors that are connected in series.
Information copied from http://www.physicsclassroom.com/class/circuits/Lesson-4/Series-Circuits Links to an external site.on December 9, 2014
Two or more electrical devices in a circuit can be connected by series connections or by parallel connections. When all the devices are connected using parallel connections, the circuit is referred to
as a parallel circuit. In a parallel circuit, each device is placed in its own separate branch. The presence of branch lines means that there are multiple pathways by which charge can traverse the external circuit. Each charge passing through the loop of the external circuit will pass through a single resistor present in a single branch. When arriving at the branching location or node, a charge makes a choice as to which branch to travel through on its journey back to the low potential terminal.
A short comparison and contrast between series and parallel circuits was made in an earlier section of Lesson 4 Links to an external site.. In that section, it was emphasized that the act of adding more resistors to a parallel circuit results in the rather unexpected result of having less overall resistance. Since there are multiple pathways by which charge can flow, adding another resistor in a separate branch provides another pathway by which to direct charge through the main area of resistance within the circuit. This decreased resistance resulting from increasing the number of branches will have the effect of increasing the rate at which charge flows (also known as the current). In an effort to make this rather unexpected result more reasonable, a tollway analogy Links to an external site. was introduced. A tollbooth is the main location of resistance to car flow on a tollway. Adding additional tollbooths within their own branch on a tollway will provide more pathways for cars to flow through the toll station. These additional tollbooths will decrease the overall resistance to car flow and increase the rate at which they flow.
Current
The rate at which charge flows through a circuit is known as the current Links to an external site.. Charge does NOT pile up and begin to accumulate at any given location such that the current at one location is more than at other locations. Charge does NOT become used up by resistors in such a manner that there is less current at one location compared to another. In a parallel circuit, charge divides up into separate branches such that there can be more current in one branch than there is in another. Nonetheless, when taken as a whole, the total amount of current in all the branches when added together is the same as the amount of current at locations outside the branches. The rule that current is everywhere the same still works, only with a twist. The current outside the branches is the same as the sum of the current in the individual branches. It is still the same amount of current, only split up into more than one pathway.
In equation form, this principle can be written as
Itotal = I1 + I2 + I3 + ...
where Itotal is the total amount of current outside the branches (and in the battery) and I1, I2, and I3 represent the current in the individual branches of the circuit.
Throughout this unit, there has been an extensive reliance upon the analogy between charge flow and water flow. Once more, we will return to the analogy to illustrate how the sum of the current values in the branches is equal to the amount outside of the branches. The flow of charge in wires is analogous to the flow of water in pipes. Consider the diagrams below in which the flow of water in pipes becomes divided into separate branches. At each node (branching location), the water takes two or more separate pathways. The rate at which water flows into the node (measured in gallons per minute) will be equal to the sum of the flow rates in the individual branches beyond the node. Similarly, when two or more branches feed into a node, the rate at which water flows out of the node will be equal to the sum of the flow rates in the individual branches that feed into the node.
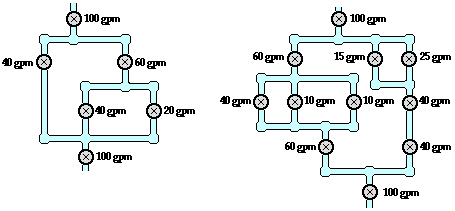
The same principle of flow division applies to electric circuits. The rate at which charge flows into a 
node is equal to the sum of the flow rates in the individual branches beyond the node. This is illustrated in the examples shown below. In the examples a new circuit symbol is introduced - the letter A enclosed within a circle. This is the symbol for an ammeter - a device used to measure the current at a specific point. An ammeter is capable of measuring the current while offering negligible resistance to the flow of charge.
Diagram A displays two resistors in parallel with nodes at point A and point B. Charge flows into point A at a rate of 6 amps and divides into two pathways - one through resistor 1 and the other through resistor 2. The current in the branch with resistor 1 is 2 amps and the current in the branch with resistor 2 is 4 amps. After these two branches meet again at point B to form a single line, the current again becomes 6 amps. Thus we see the principle that the current outside the branches is equal to the sum of the current in the individual branches holds true.
Itotal = I1 + I2
6 amps = 2 amps + 4 amps
Diagram B above may be slightly more complicated with its three resistors placed in parallel. Four nodes are identified on the diagram and labeled A, B, C and D. Charge flows into point A at a rate of 12 amps and divides into two pathways - one passing through resistor 1 and the other heading towards point B (and resistors 2 and 3). The 12 amps of current is divided into a 2 amp pathway (through resistor 1) and a 10 amp pathway (heading toward point B). At point B, there is further division of the flow into two pathways - one through resistor 2 and the other through resistor 3. The current of 10 amps approaching point B is divided into a 6-amp pathway (through resistor 2) and a 4-amp pathway (through resistor 3). Thus, it is seen that the current values in the three branches are 2 amps, 6 amps and 4 amps and that the sum of the current values in the individual branches is equal to the current outside the branches.
Itotal = I1 + I2 + I3
12 amps = 2 amps + 6 amps + 4 amps
A flow analysis at points C and D can also be conducted and it is observed that the sum of the flow rates heading into these points is equal to the flow rate that is found immediately beyond these points.
Equivalent Resistance
The actual amount of current always varies inversely with the amount of overall resistance. There is a clear relationship between the resistance of the individual resistors and the overall resistance of the collection of resistors. To explore this relationship, let's begin with the simplest case of two resistors placed in parallel branches, each having the same resistance value of 4 Ω. Since the circuit offers two equal pathways for charge flow, only one-half the charge will choose to pass through a given branch. While each individual branch offers 4 Ω of resistance to any charge that flows through it, only one-half of all the charge flowing through the circuit will encounter the 4 Ω resistance of that individual branch. Thus, as far as the battery that is pumping the charge is concerned, the presence of two 4-Ω resistors in parallel would be equivalent to having one 2-Ω resistor in the circuit. In the same manner, the presence of two 6-Ω resistors in parallel would be equivalent to having one 3-Ω resistor in the circuit. And the presence of two 12-Ω resistors in parallel would be equivalent to having one 6-Ω resistor in the circuit.
Now let's consider another simple case of having three resistors in parallel, each having the same resistance of 6 Ω. With three equalpathways for charge to flow through the external circuit, only one-third the charge will choose to pass through a given branch. Each individual branch offers 6 Ω of resistance to the charge that passes through it. However, the fact that only one-third of the charge passes through a particular branch means that the overall resistance of the circuit is 2 Ω. As far as the battery that is pumping the charge is concerned, the presence of three 6-Ω resistors in parallel would be equivalent to having one 2-Ω resistor in the circuit. In the same manner, the presence of three 9-Ω resistors in parallel would be equivalent to having one 3-Ω resistor in the circuit. And the presence of three 12-Ω resistors in parallel would be equivalent to having one 4-Ω resistor in the circuit.
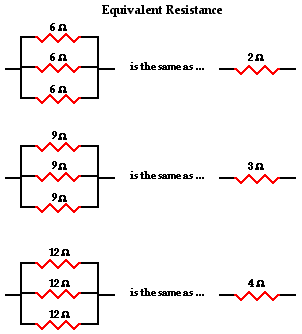
This is the concept of equivalent resistance. The equivalent resistance of a circuit is the amount of resistance that a single resistor would need in order to equal the overall effect of the collection of resistors that are present in the circuit. For parallel circuits, the mathematical formula for computing the equivalent resistance (Req) is
1 / Req = 1 / R1 + 1 / R2 + 1 / R3 + ...
where R1, R2, and R3 are the resistance values of the individual resistors that are connected in parallel. The examples above could be considered simple cases in which all the pathways offer the same amount of resistance to an individual charge that passes through it. The simple cases above were done without the use of the equation. Yet the equation fits both the simple cases where branch resistors have the same resistance values and the more difficult cases where branch resistors have different resistance values. For instance, consider the application of the equation to the one simple and one difficult case below.
|
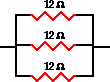
Case 1: Three 12 Ω resistors are placed in parallel
|
1/Req = 1/R1 + 1/R2 + 1/R3
1/Req = 1/(12 Ω) + 1/(12 Ω) + 1/(12 Ω) Using a calculator ... 1/Req = 0.25 Ω-1 Req = 1 / (0.25 Ω-1) Req = 4.0 Ω
|
|
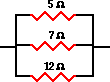
Case 2: A 5.0 Ω, 7.0 Ω, and 12 Ω resistor are placed in parallel
|
1/Req = 1/R1 + 1/R2 + 1/R3
1/Req = 1/(5.0 Ω) + 1/(7.0 Ω) + 1/(12 Ω) Using a calculator ... 1/Req = 0.42619 Ω-1 Req = 1 / (0.42619 Ω-1) Req = 2.3 Ω |
Information copied from http://www.physicsclassroom.com/class/circuits/Lesson-4/Parallel-Circuits Links to an external site.on December 9, 2014
Qucs Part 2 Drawing a Simple Schematic and DC Analysis Simple Circuits
Links to an external site.
Laplace Domain Circuit Analysis Simple Circuits
Links to an external site.
Electric Circuit Fundamentals: Components and Types Links to an external site. (video with quiz and lots of great information on this site)
Suggested Objective d: Analyze and explain the relationship between magnetic fields and electrical current by induction, generators, and electric motors
We will participate in several assignments and projects that will assist you with learning about magnetism, electric fields, and electricity. Keep your notes handy and add to them as we proceed through our activities. Let's begin!